
- #Derive electric flux formula how to
- #Derive electric flux formula update
- #Derive electric flux formula free
This allows you to learn about Electrostatics and test your knowledge of Physics by answering the test questions on Electrostatics. At the end of each Electrostatics tutorial you will find Electrostatics revision questions with a hidden answer that reveals when clicked.
#Derive electric flux formula how to
Each Electrostatics tutorial includes detailed Electrostatics formula and example of how to calculate and resolve specific Electrostatics questions and problems.
#Derive electric flux formula free
The following Physics tutorials are provided within the Electrostatics section of our Free Physics Tutorials. We believe everyone should have free access to Physics educational material, by sharing you help us reach all Physics students and those interested in Physics across the globe.Įlectrostatics Physics Tutorials associated with the Electric Flux Calculator

This allows us to allocate future resource and keep these Physics calculators and educational material free for all to use across the globe. It is also sometimes necessary to do the inverse calculation (i.e., determine electric field associated with a charge distribution). where D is electric flux density and S is the enclosing surface. For convenience we assume that 0 at t 0, which implies R t and S L R. The integral form of Gauss’ Law is a calculation of enclosed charge Qencl using the surrounding density of electric flux: SD ds Qencl. Derivation via the Divergence Theorem Equation 5.7.2 may also be obtained from Equation 5.7. Induction Motor Equations ENGN1931F Spring 2017 2 Let L R S and be the angular velocities of the magnetic field (line frequency), rotor, and slip respectively. We hope you found the Electric Flux Calculator useful with your Physics revision, if you did, we kindly request that you rate this Physics calculator and, if you have time, share to your favourite social network. Gauss’ Law in differential form (Equation 5.7.2) says that the electric flux per unit volume originating from a point in space is equal to the volume charge density at that point. You can then email or print this electric flux calculation as required for later use.
#Derive electric flux formula update
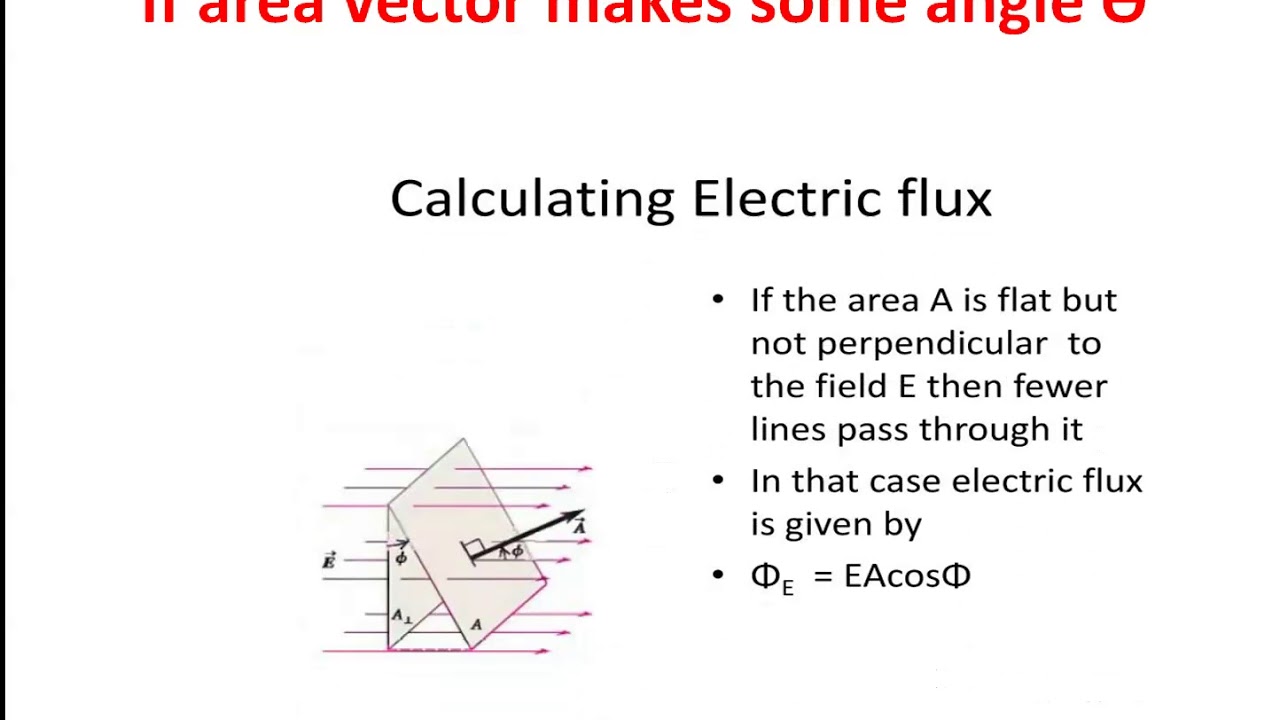
As you enter the specific factors of each electric flux calculation, the Electric Flux Calculator will automatically calculate the results and update the Physics formula elements with each element of the electric flux calculation. Please note that the formula for each calculation along with detailed calculations are available below. Surface area Note 1 ( |A|) m 2 Īngle between electric field lines and the area vector ( θ) ° Įlectric constant or vacuum permittivity ( ϵ 0) C 2/N∙m 2 The electric flux through a closed surface when the charge is given using the Gauss Law isĮlectric flux calculations for inward fluxĮlectric flux calculations for outward flux The electric flux (outward flux) through a closed surface when electric field is given is V ∙ m This alternative description offers some actionable insight, as we shall point out at the end of this section. Electric Flux (Gauss Law) Calculator Results (detailed calculations and formula below) The electric flux (inward flux) through a closed surface when electric field is given is V ∙ m Electric flux density, assigned the symbol, is an alternative to electric field intensity () as a way to quantify an electric field.


 0 kommentar(er)
0 kommentar(er)
